Chess puzzles

It is no surprise that the game of chess generates such an amount of mathematical puzzles. The amount of riddles of combinatoric nature alone should have the readers busy for a long while, no matter what their chess strength is. This is a selection of several puzzles designed with that purpose in mind.

Gyozo Nagy’s “Expensive Armies”

It is well known that exactly 8 queens can be placed on a chessboard without attacking each other; similarly 8 rooks, or 14 bishops, or 32 knights, or 16 kings. But, what if we are allowed to mix pieces?

Let us assign values of \( \frac{1}{8} \) to a queen; \( \frac{1}{8} \) to a rook; \( \frac{1}{14} \) to a bishop; \( \frac{1}{32} \) to a knight; and \( \frac{1}{16} \) to a king.

• Construct the most expensive army on a chessboard, using any number of these pieces, so that no piece attacks another. (Here “any number” includes zero.)
• Same thing, but disallowing kings (so our army consists only of queens, rooks, bishops and knights).

Can these mixed armies achieve values greater than one? In each case give the largest possible value (and a setup achieving it).

For example, the setup on the board below has an army value of

I found this problem in August 2003 “Ponder This” challenge in the pages of research.ibm.com. They offer a couple of solutions (included in the diagram below), and a note indicating that some puzzle solvers used integer programming to prove optimality of those. Note that neither solution uses a single queen.

The diagram on the left shows an example for the first part of the puzzle, with an army of value

The diagram on the right shows an example for the second part of the puzzle, with an army of value

Martin Gardner’s “Minimum and Maximum Attacks”

I found these problems in Martin Gardner’s “The Unexpected Hanging and Other Mathematical Diversions.” They were posed in the eighteen hundreds, and some variations are still open for optimal solution.

The minimum-attack problem. Place the eight pieces of one color (king, queen, two bishops, two knights, two rooks) on the board so that the smallest possible number of squares are under attack. A piece does not attack the square on which it rests, but of course it may attack squares occupied by other pieces.

The maximum-attack problem—weak version. Place the same eight pieces on the board so that the largest possible number of squares are under attack. In the weak version, we do not force both bishops to be on opposite colors.

The maximum-attack problem—strong version I. As before, but forcing the two bishops to be on opposite colors.

The maximum-attack problem—strong version II. As in strong version I, but forcing the king into a non-attacked square!

Husserl’s “Third-man theme”

Notice on the board below, the two sole occupants are the kings of opposite sides. Add a third piece, creating a position that satisfies the following conditions:

1. Neither king is in check.
2. The position can be reached in a legal game.
3. It can be proved that neither side has a legal play

Note the subtlety of the puzzle: we are searching not only for a double stalemate, but one in which neither side can move! The solution happens to be unique.

Miscellaneous

All the diagrams in this page are generated in \( \LaTeX \) with the package skak, by using the command \fenboard and the Forsyth–Edwards notation. For example, the first diagram above has the (very simple) code

1
2
3
\newgame
\fenboard{4Q3/R7/3R4/6N1/7R/1K6/5K2/2R5 w - - 0 0}
\showboard

The string in the command \fenboard is to be interpreted as follows:

• 8-th row: start with four empty squares, then place a white queen (Q), followed by three empty squares.
• 7-th row: a white rook (R), followed by seven empty squares.
• 6-th row: three empty squares, one white rook, four empty squares.
• and so on, until the first row, that reads: two empty squares, then a white rook followed by five empty squares.
• The remaining commands (which are optional) indicate that white is to move (w), that there are no castling options for neither side (-), no en passant squares (-) no half-moves since the last capture (0) and no move number (0).

The skak package is originally designed to create both an annotated game and its corresponding diagram. For example, the following annotation of a variant of the Ruy Lopez opening first moves is generated with the simple list of commands included:

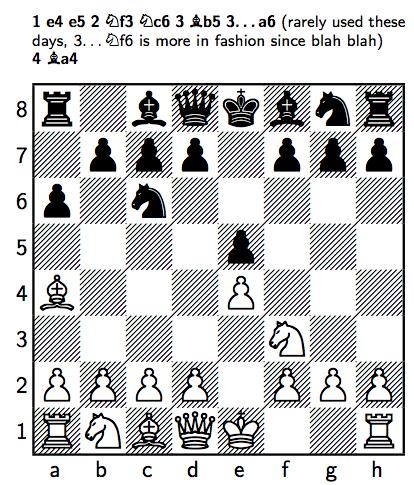1 2 3 4 5 6 7 8 9 10 11 \newgame \mainline{1.e4 e5 2.Nf3 Nc6 3.Bb5} \mainline{3...a6} (rarely used these days, \variation{3...Nf6} is more in fashion since blah blah) \mainline{4.Ba4} \vspace{0.25cm} \showboard